An Important Property of Every Line Is Its Steepness or

Slope is a way of describing the steepness of an object. MPt MPc MPt.

Slope Of A Line Beginning Algebra

It simply indicates how much the line rises per unit move to the right or how much it goes down as we move to the right.

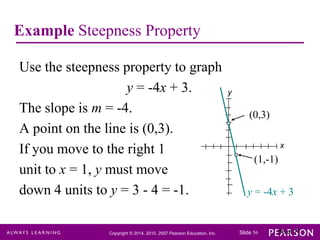
. So the slope of the budget line is. It is given by the increase in the vertical coordinates divided by the increase in the horizontal coordinates. One property of a line is its slope which is a measure of the steepness of the line.

In math slope is used to describe the steepness and direction of lines. For simplicity k s A 0 m n can also be denoted U a dimensionless value that represents relative uplift rate without necessitating an estimate of mn. When the numbers of wins and losses are increased by a factor of five Fig.

The product of a number and its reciprocal equals 1. Riserun 16-45-1 124 3. Firstly the slope of a line is an idea so much more broad and applicable than it sounds - the maths of it is not limited to physical steepness like a steep road although this is a useful starting point and gives our brains something physical to hang the concept on.

4b the P ij s remain unchanged. Qc and there Qc MPc. Find k for a line that passes through the points 0 5 and k 20 if the slope is -4.

The gradient of each line is equal to the channel steepness index divided by the reference area raised to the power of θ or mn. How to find the slope of a line when given a graph of a line. At 4 pm the temperature was 16 degrees and at 8pm it was 4 degrees.

If the consumer buy only tea the equation becomes M P t. Slope often is used to describe the steepness of the grounds surface. Every line has a slope defined by rise over run ie the amount the line rises vertically over the amount the line runs horizontally.

It has no steepness and neither goes up nor down. So with NormDS using P ij the steepness would be considered to be high whereas with NormDS using D ij it is much lower. When given a graph of a line we need to know a simple definition of slope.

Later in this section you will learn how to determine the number representing the slope of a line. Identify slope from a graph. Slope The steepness of a line expressed as a ratio using any two points on the line.

Q t 0 and the maximum amount of tea that he can buy is. Up to 24 cash back b. A linear relationship between time and temperature yields the following data.

The slope is exactly the same as the steepness if the line goesfrom bottom-left to top-right and it is the negative value of thesteepness if the line goes from top-left to. If we increase the rise the slope also increases and if we decrease the rise the slope also decreases. The most important property of perpendicular lines is as follows.

Q t P c. The slope of a line is a number that describes how steep the line is. One of the most important properties of a line is its slope.

A line passes through the points 110 and 67 c. The steepness of a line expressed as a ratio using any two points on the line. The steeper the slope the faster the rate of change What can you determine about the steepness of.

A line with a small slope such as 010 is very flat. Many geographic information systems GIS can analyze digital elevation data elevation points contour lines digital. If either the denominator or numerator is negative the fraction is considered a negative fraction.

If both the denominator and the numerator are negative the fraction is considered to be a positive fraction. This quiz is designed to assess if you know how to find a slope given two points on a line. The steepness of the line is the slope of the line which is the rate of change.

Slope of line connecting two points. That is the speed with which the object is moving towards or away from the origin. The slope of a line is a measure of its steepness.

More precisely it would be the absolute value of the gradient since the question does not distinguish between. If we increase the run the slope decreases and if we decrease the rise. Similarly if the consumer buys only coffee the budget equation becomes M 0 Pc.

The steepness of the line on a distance-time graph represents the radial speed of the object. Consequently any line with a slope of 3 will be parallel with the line that connects 1 4 and 5 16. The Slope of a Line As we graph lines we will want to be able to identify different properties of the lines we graph.

Answer 1 of 3. The y-intercept is the base level elevation z x b. A line with a large slope such as 25 is very steep.

A horizontal line is perfectly flat. Adding two fractions of the same sign either positive or negative gives an answer. M Slope is the ratio of a line ˇs _____ change to its _____ change.

The slope of a line also describes the general direction of a. An important property of every line is its steepness or _____. It can be positive if the line slopes upwards as we look from left-to-right or it can be negative if the line slopes downhill from left to right.

The _____ is the vertical distance between two points. M P t. You will also be assessed on steepness of a given slope and.

Slope can be measured as the rise the increase in elevation in some unit of measure over the run the horizontal distance measured in the same units as the rise. The equation of the budget line is. Special building materials are used for low-slope roofs so a construction worker must know the slope of the roof before beginning a shingling project.

SLOPE A number that describes the steepness or slant of a line. That ˇs what we mean by rise over run. The mathematical definition of slope is very similar to our everyday one.

We have the following facts. Q t MP t. By just looking at the graph of a line you can learn some things about its slope especially relative to other lines graphed on the same coordinate plane.

Its gradient or slope. A line passes through the points and d. Slope is very important in the construction field because it often dictates the best way to complete a project.

Slope is a measure of steepness. One of the most important properties of a line is its slope. You can compute the slope of a line by using the slope _____.

The steepness of the fitted line is 092 for NormDS using P ij and 052 for NormDS using Dij Fig. The slope of a line is its angle or steepness. The slope is a measure of the steepness of a line It is a ratio between vertical change run and horizontal change rise so we can also write the slope m as.

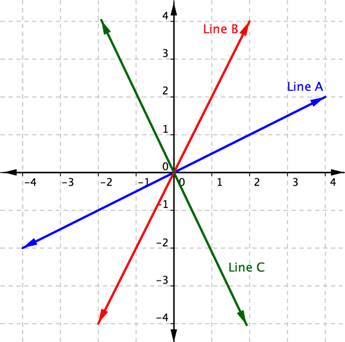
Line A is perpendicular to Line B if Line A intersects Line B at a 90 angle.

Chapter 1 Linear Equations And Straight Lines

Chapter 1 Linear Equations And Straight Lines

Finding The Steepness Of A Line Ck 12 Foundation

Finding The Steepness Of A Line Ck 12 Foundation